-
Posts
6975 -
Joined
-
Last visited
-
Days Won
66
Content Type
Profiles
Forums
Events
Gallery
Blogs
Everything posted by bonanova
-
2n distinct points in the plane, no three of which are collinear, are colored red or blue in equal numbers. Is there a red-blue paring of the points that permits the pairs to be joined by n line segments with no crossings?
-
Six line segments serve to connect pairwise any four points in the plane, no three of which are collinear. It's clear that no placement of the points permits all six to have the same length. How many unique placements permit the segments to have only two distinct lengths? Example:
-

Green and Yellow hats again, but harder
bonanova replied to bonanova's question in New Logic/Math Puzzles
In this puzzle, they're not in a line. -

Green and Yellow hats again, but harder
bonanova replied to bonanova's question in New Logic/Math Puzzles
In games of this type every guess, on average, is wrong 50% of the time. For the 3-prisoner strategy, if we count the number of guesses made in all 8 cases, we find six of them are correct and six are incorrect. We "rescue" the other cases, (when both colors are seen, and no guidance is available,) with the "Pass" option. The same is true in the previous G-Y puzzle. The difference is that in the first puzzle every wrong guess produced a fatality, so survival also was only 50%. In the 3-prisoner case here, death uses up three wrong guesses (while survival still requires only a single correct guess.) We "packed" the incorrect guesses into the fewest possible of the eight cases and thus raised survival to 75%. But since 2x3 = 6x1 randomness still got its due. The 3-prisoner solution is trimmed-down version of the n-prisoner strategy. Have fun... -
As promised, a harder hat problem. Prisoners are seated in a circle so they can see all the others. This time the warden flips a fair coin for each prisoner and gives him a yellow or green hat, accordingly. Once all the hats have been placed, and have been seen by the others, prisoners are taken aside singly and given the opportunity to guess the color of his hat. And if instead he chooses not to guess, he is permitted to pass. Now comes the bad part. Unless at least one prisoner guesses, and all the prisoners who do guess are correct, all the prisoners will be executed. That's right, survival requires perfection from every prisoner who guesses his color. Prisoners decide on a strategy beforehand, and after the first hat is placed there is no further communication. Clearly, there can be a single "designated guesser" who ... just ... guesses a color. Half the time they all survive. But what kind of a puzzle would that be? Yes, incredibly, the prisoners can do much better. How? Maybe thinking about a three-prisoner case will answer that question. Once you're convinced they can do better than a coin toss, find their best strategy.
-
Have fun with this one. A bull stands in a pasture, unaware that he has just swallowed a time bomb that is due to explode in five minutes. Which word best describes the situation? Awful Abominable Dreadful Shocking Five minutes have passed, and all that's visible from the above scene is a sizable hole in the ground and scraps of bone and flesh. Which word best describes this situation? Amazing Silly Messy Noble
-
What shall I do, Gerry? Asking nutty questions can be most annoying A gold key is not a common key. Horace tries in school to be a very good boy. People who drive too fast are likely to be arrested. Did I ever tell you, Bill, I once found a dollar? John came late to his arithmetic class. I enjoy listening to music at night.
-
Can you write down a 9-letter word that permits you to erase it, one letter at a time, such that after each erasure a valid (English) word remains? (As implied, the letter order remains the same throughout.) Clue: Example:
-
Here are nine words before, after, and in-between which only one word can be placed that gives ten different meanings to the sentence. The word is used only once, in only one space at a time, to obtain the ten different meanings. What's the only word that works? _____ TOM _____ HELPED _____ MARY'S _____ DAUGHTER _____ CLEAN _____ MARY'S _____ PARROT'S _____ CAGE _____ YESTERDAY _____.
-
@Molly Mae Fair question.
-
Yay! And does it seem strange to anyone else that something 7% the size of the circle on average covers the center 25% of the time? (My red herring was soooo totally ignored. Good job for doing that.)
-
Ah!
-
Kudos to all. @flamebirde set up an attack framework, @Izzy got the puzzle to its knees, @Molly Mae knocked it out for the count and finally @plasmid, who just might have a penchant for shooting rabbits with elephant guns, put a bullet through its head. To sort out the credits I'll don my judge's robes and presume to declare a verdict: @flamebirde told us it's all about angles. (Honorable mention. +1) @Molly Mae made four (equal) quadrants, which I have in my solution, and gave the right answer but didn't prove it, then switched to the @flamebirde - @Izzy model and backed up the answer with a word proof. (FTW) What must have been only moments later, @Izzy put numbers on things but, probably exhausted from her class, got the wrong number. (Honorable mention. +1) @plasmid dazzled us with calculus symbols and cold-blooded math to lay the newly-deceased puzzle to rest. (also Honorable mention, +1 but it doesn't seem enough. Timing is everything.) As I followed your discussion, (credit to you all) it occurred to me that this might be the simplest non-math approach:
-
@Molly Mae and @Izzy you both
-
Right so far, and the one not yet calculated is interesting.
-
This approach saves |n/2| prisoners, and that is optimal.
-

Jelly beans join the clean plate club
bonanova replied to bonanova's question in New Logic/Math Puzzles
That's it. Binary representation of B/A indicates moves to make B smaller than A was. Rinse and repeat. Nice. -
OK so I see MM quoting an Izzy post, but I don't see that original post. Can I invite Izzy to repost it, with complete description? Because ... it's the solution. Nice solve.
-
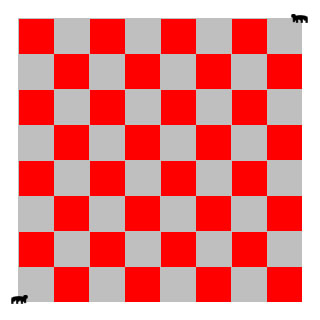
Two ants named Al and Bert sit at diagonal corners of a checkerboard and decide to change places. Al, at the lower left, walks randomly upward or to the right, and Bert, at the upper right, walks randomly downward or to the left. They follow the boundaries of the checkerboard squares. That is, except when following the extreme boundary of the checkerboard, their left and right feet always touch squares of opposite color. What is the probability of their meeting (1) if they walk at the same speed, or (2) if Al walks 3 times as fast as Bert?
-
Red herring (which I think you've figured out)
-
Hint:
-
Not sure if ... answer is in the title Sell to? Yes. I fixed the OP. Good catch. When I see ... right track.
-
Place two dimes and two pennies in a line with a space between them like this: P P D D _ _ _ _ _ Using a sequence of moves, switch the two groups of coins to achieve this position: D D P P _ _ _ _ _ Moves are of two types: slide a coin to an (adjacent) empty space. jump a coin over another coin into an empty space. Type (1) move: P P D D _ _ _ _ _ Type (2) move: P D P D _ _ _ _ _ The underlines show the (only) five legal locations for the coins to occupy. What is the smallest number of moves needed?
-
The word buried here has but one letter. Did you find a jelly roll in Gearhardt's Bakery? It's the best one I've ever seen. The rug at her stairway was made in India. He's an old friend. Amos sold his bicycle to an old friend.