8/15

It never will! For 2n+2 blades, the probablility of a loop is Product{i=1 to n}(2i/(2i+1)). This product has all odd factors in its denominator. Since there are never any 2s in the factorization of the denominator, the fraction could never simplify to 1/8.

The closest that the probability gets to 1/8 is with 110 blades, having a loop probability of approximately .11977.

According to folklore, marital fortunes were once told by a simple method.

A girl would hold six long blades of grass in her closed hand, the blades protruding from each side.

Another girl would tie the ends in pairs, at random, first on one side, then on the other.

The first girl would then open her hand and inspect how the blades were connected.

The possible outcomes are three small loops; one small loop and one medium loop; and one large loop.

If the blades became one large loop, the girl doing the tying would be married within the year!

The puzzle is in two parts:

.

1. What is the probability of obtaining one large loop?
2. How many blades of grass must the first girl hold for the probability to fall to 1/8?

.

As always, enjoy!

8/15

It never will! For 2n+2 blades, the probablility of a loop is Product{i=1 to n}(2i/(2i+1)). This product has all odd factors in its denominator. Since there are never any 2s in the factorization of the denominator, the fraction could never simplify to 1/8.

1) ~1.216

2)a square antiprism (basically a cube with 2 opposing faces twisted 45 degrees relative to each other)

It would have 10 faces; 8 of them triangles, 2 of them squares

Matou's got it!

If I ask you to place N equally space points on a circle,

you would have no particular trouble doing so. The same

is not true for equally spaced points on a sphere, as is

evidenced by the irregular-looking pattern of dimples

on a golf ball.

But what does equally spaced points on a sphere mean?

I have come up with a working definition which is probably

not original to me: The points are equally spaced if and

only if the minimum distance from a point to any other

point is maximized. That is, find the closest neighbor

to every point, then find the smallest such distance,

and make sure that it is as large as possible. So, for

a 2-point example, the points would be antipodal -- that's

as far away from each other than they can get. For three

points, they will all lie on a great circle. For four

points, they form the vertices of a regular tetrahedron

(a tetrahedron of which all edges have the same length).

Suppose one places 8 equally-spaces points (according to

the definition above) on a unit sphere (a sphere of radius

1). What would be the shortest euclidean distance between

any pair of points? Euclidean distance is the straight-line

distance (not the distance over the surface of the sphere)

between the points. How would you describe the figure

whose vertices are those points?

It is NOT a cube.

It is easy to prove that, if you need k more toys, the expected wait to get at least one of them is 10/k. All you need to do is a bit of algebraic manipulation on an infinite convergent power series. So, the exact answer *really* is (10/10)+(10/9)+(10/8)+...+(10/1).

Okay I think I got it now:

A small mistake leads to a big error in the final answer, as you know.

So far, we have essentially 5 different answers to this problem in

this forum. Lots of people are making lots of different mistakes!

I also got around 62" as the answer. You were very quick to recover from your error. By the way, I couldnt fit the ladder in the shed because the wall was only 48" high before it hit the A-frame of the shed roof.

The length of the ladder is the hypotenuse (193 inches), but the base of the

triangle is a bit smaller than 192 inches because it ends where it touches the

bottom edge of the ladder, at the vertical line segment on the right side of

your graphic. So the base is 192-y inches for some y.

Wow! Three essentially different calculated answers!

Did anyone try to make a scale model of the problem

out of paper to get a ballpark on this? I'll say one

thing: The off-the-cuff guesses some of you made were

all closer to the truth than my original guess.

Thanks for your interest in this problem.

This problem actually came up in real life for me.

This is definitely not a trick question. So, if

there's something you don't understand about the

problem statement, I'll be happy to clear it up.

I was trying to store a ladder in a shed.....well,

you'll see:

I would like to store a ladder inside a shed.

I want to store it flat against the wall using hooks.

The shed wall is 16 feet long (192 inches). The

ladder is 16 feet and 1 inch long (193 inches)

and it is 18 inches wide. What is the minimum

height that the shed wall must be to allow me to

mount the ladder flat against this wall without

poking through the floor, ceiling, or side walls?

My shoot-from-the-hip initial guess was way off.

So, I was surprised at the height needed after

I worked it out. I would ask the solver to give

2 answers: (1) An initial guess and (2) The

actual value (to the nearest inch or so).

I would be particularly interested in an "AHA!"

solution.

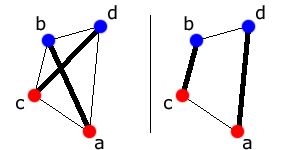
Take all the possible pairings of the red and blue points.

For one of the pairings of all the points, the total length of the connecting line segments will be smallest.

Call this the minimal-length pairing [MLP].

This pairing has no crossings.

Proof: assume the MLP has a crossing.

Specifically, suppose that in the MLP, the segment connecting a to b crosses the segment connecting c to d.

Since no 3 points are collinear, adbc is a quadrilateral with [crossing] diagonals ab and cd.

By the triangle inequality we can reduce the total length by pairing a with d and c with b.

Thus the MLP was not the MLP; that is, the assumption is disproved.

More discussion

Oops! I even searched to see if I could find this puzzle here.

I guess that shows how poor a searcher I really am!

Sorry to have duplicated a puzzle.

Suppose we have 2N points in the plane. N of the points

are Red and N of them are Blue. Furthermore, no subset of

3 of these 2N points lie along a line.

Prove or disprove:

It is always possible to pair up the Red and Blue

points into N pairs, one Red and one Blue in each pair

connected by a line segment, in such a way that no line

segment crosses another line segment.

I think this is optimal for the square:

The best I get has area 9/8 and edge length .75*sqrt(2) as follows: From a corner on one face place two corners of the square a distance d away from that corner along the two edges of that face that form the corner. So, the edge length will be d*sqrt(2). Next we go to the corner spatially diagonal to the corner we started from and place the two corners for the square d away from the cube corner on the face opposite our starting face. We calculate the spatial edge of the square to be sqrt(2*(1-d)^2+1) because the two spatial end points (The way I places the square in the coordinate system) are ((1,1,d) and (d,0,1). So, to be a square, the spatial edges must equal the facial edges. So, d*sqrt(2)=sqrt(2*(1-d)^2+1). solving for d, we get .75. The square, then, has edge .75*sqrt(2). The area is 9/8.

An intriguing problem, indeed. I have some thoughts

which may be of some help in approaching it:

If we drop down a dimension to the unit interval, one would hope that the problem would be a lot easier. Even though we are now dealing with lengths instead of areas, it still seems to be quite difficult. Discussion of this one-dimensional case may prove fruitful. I thought of this because there exist one-to-one functions between the unit interval and the unit square (although they can't be continuous).

Technically, at any flip, it is guaranteed that your number will either be greater than 1/pi or less than 1/pi. The proper early stopping conditions should be if your binary number X is greater than 1/pi, or if X is less than 1/pi regardless of subsequent flips. The second condition makes it messy to calculate the average required number of flips, so Im just going to go out on a limb and estimate the expected number of flips as 1 + 1/2 + 1/4 + 1/8 ... = 2 flips.

Ok, I wasn't rigorous enough in my reply, Mea Culpa!

This will guarantee any desired degree of accuracy

Let heads = 1, let tails = 0. Imagine that every time you flip a coin, you are adding binary digit to a fractional binary number.

So, suppose you flipped head, tail, head, head, that would correspond to the binary number .1011_b, which, in decimal notation is equal to 1/2 + 0/4 + 1/8 + 1/16 = 11/16 = .6725. Suppose we start over and flip tail,tail,head, head, that would correspond to .0011_b in binary, which is .1875 in decimal.

So, 1/pi is 0.3183099 in decimal. To satisfy the OP, let the two mathematicians flip the coins and construct the fractional binary number, call it X. Let the two agree on a predetermined number of flips n. After n flips, convert X to a decimal number. If it is less than 1/pi, then guy A buys guy B a beer. If it is greater than 1/pi, no free beer. You can improve the accuracy of this method by increasing the number of flips. This method is related to the bisection method. The maximum error, or the difference in the produced probability and the threshold, is 1/( 2ⁿ)

This method does not require the full n flips. At any flip, if the number X is greater than the threshold regardless of subsequent flips, then we might as well stop. For instance, for the threshold 1/pi = .31, if the first flip is head, then the number X is guaranteed to be greater than .5, so we might as well stop.

This also works for any arbitrary threshold besides 1/pi.

Yes! But, in practice you don't really have to agree on an n, after all the, likelihood that n gets big enough to be a pain if you let it grow is very very small. So, just keep flipping until you either get smaller or larger than 1/pi.

But, what about the second part of the question? If you keep flipping until the matter is decided, what is the expected number of flips?

I should have added that the method should work

for any probability -- not just 1/pi.

Check out Buffon's needle.

Draw parallel lines on the table, separated by exactly the diameter of the coin.

Draw a line across the diameter of the coin (one on each side, doesn't matter if they are parallel).

Flip the coin. The coin's line will touch or cross a table line with probability 2/pi.

To scale this down to 1/pi, flip twice. If it comes up heads both times or tails both times, buy the beer. If different, no beer.

AH! But to be exact, the lines would have to have no thickness.

Ya can't do that!

This is not a trick question. It is indeed possible to

use the results of ordinary coin flips to decide something

with probability exactly 1/pi. "any required accuracy"

means exactly what it says, so Fred and Mike could determine

any decimal place, no matter how far out it is, if they

required it.

Okay, if I'm understanding this correctly, there is a one in pi chance that Mike is getting a free beer.So if you simply divide 1 by pi, you get approx. 32%. So how do you get 32% from flipping coins? I'll get back to you

No "approximately", we're talking "exactly" here!

Fred and Mike, two mathematicians, are in a bar.

Fred suddenly feels magnanimous and offers to buy Mike

a beer with probability 1/pi. As good mathematicians,

they would have no trouble calculating 1/pi to any

required accuracy. They agree to decide the matter

using only the results of flipping a fair coin some

number of times. How can they do this? And, on

average, how many coin flips would be required to

decide the matter? Of course, minimizing the number

of average coin flips would be important to them.

Can we potentially reduce the loops by realizing that as N repeats, so does floor(N/60) ???? I was looking for potential bottom up solutions with respect to this earlier but ran out of time...

tpaxatb asked why I chose the particular numbers in the puzzle:

I picked 123456789 because it was easy to remember. I chose the other numbers

using a little theory about linear congruential generators and a bit of fiddling

in order to get a relatively small cycle with a rather large tail leading

into it.

By the way, none of the algorithms posted here are general enough to work in

all cases; cycles on the order of trillions would be missed by all of them.

There are ways to detect cycles which use very little space, are quite fast

(i.e., the running time is linear in the length of the cycle), and are

completely general. You can find them out there on the web.

I wrote a quick monte carlo program which

played the game 100,000,000 times. I get:

Russia wins 16.97% of the time; Ties (both lose) 5.1% of the time; Loses 77.93% of the time.

Oops, I gave the program incorrect data.

19.685% of the time Russia wins; 7.234% of the time there's a tie; 73.081% America wins

Determine all possible nonzero real n satisfying n = [n]*{n}, such that 5*{n} - [n]/4 is an integer.

Note: [x] is the greatest integer <= x, and {x} = x -[x]

-3.2 is the only possibility. It's easy to prove but it is also a little tedious.

America; 12M 10C

Russia; 10M 14C

Common logic says that America would win, because it it America. Observant logic says that Russia would win, as they have more targets to take fire. While military logic argues that America would win, because they have more weapons. Numerical logic would agree that America would win, because they are able to emiminate more targets.

As weaponry is the only active variable, variable logic would say that America would win.

With my number generator, I got Russia winning 21.6%, Ties 2.3% and America winning 60%. Out of 1,000 trials.

Something's wrong with your numbers: They don't add up to 100%.

I wrote a quick monte carlo program which

played the game 100,000,000 times. I get:

Russia wins 16.97% of the time; Ties (both lose) 5.1% of the time; Loses 77.93% of the time.