bonanova Posted April 28, 2014 Report Share Posted April 28, 2014 (edited) Take any four points A, B, C, D in the plane, no three of which are collinear. They describe a unique quadrilateral, if we take the points as being its vertices. But they also describe a square, if we require only that the points lie on its sides. Using a compass and straightedge, construct a square such that the four points lie, one each, on its sides Edit: or the extensions of its sides. . . . . . . . . . . . . . . . Hint: The points are not special. Draw four similar points and do the construction on a sheet of paper if that helps. The answer would then be to describe the process. Edited June 6, 2014 by bonanova Relax the constraints 1 1 Quote Link to comment Share on other sites More sharing options...
0 plasmid Posted April 28, 2014 Report Share Posted April 28, 2014 (edited) Are you sure the question is phrased correctly, particularly the part about the points lying one each on the sides?Consider A (0,1), B (-1,0), C (0,-1), D (9999999,0). If each side of the square must have one point on it, then points A and C must lie on two different sides of the square. The sides with points A and C cannot meet to form a vertex, because point B must have its side lie to the left (negative x-coordinate) of line AC while point D must have its side to the right -- if the lines with points A and C were to meet at a vertex either to the right or to the left of x=0 then there could be no other lines of the square on that side of x=0. So A and C must lie on opposite sides of the square. Because those sides are parallel, the maximum distance between them is the distance between A and C which is 2. Then there's no way to have points B and D be on sides of the square because they're too far apart for a square with maximum side length 2.But there is definitely a solution for that set of points if you don't require one point per side.Edit: I can also think of an arrangement of points that can't have a square touching all four points even if you don't require one point per side. Edited April 28, 2014 by plasmid Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted April 28, 2014 Author Report Share Posted April 28, 2014 You may be right that not every set of four points will work as the OP asks. So can you construct a square on the points roughly as shown? Perhaps then derive conditions for the four points, that it can be done? This may be a richer problem that I originally thought. Quote Link to comment Share on other sites More sharing options...
0 plasmid Posted May 1, 2014 Report Share Posted May 1, 2014 For now, I'll point out some things which would render squaring the points impossible, placing some limits on the answer.First, the points must be convex and not concave. If they are concave (think of the three vertices of an equilateral triangle plus a point in the middle), meaning that there is at least one point such that any line through that point will have some of the other three points on one side of that line and some points on the other side, then it would be impossible to draw one edge of a square going through that point and have the three other edges of the square (which must all be on the same side of that original edge) touch all of the other points.Second, since the points must be convex, it is possible to define opposite points -- if you were to draw a convex quadrilateral connecting all the points then the opposite points are the pairs that do not share an edge of the quadrilateral. Those opposite points must be on opposite edges of the square because of the criteria to have one point per edge -- if two points are on adjacent edges then the other two edges must both fall on the same side of a line drawn through those two points, but a line connecting two opposite points will have a point on each side that needs to be included in the square.Since both pairs of opposite points must lie on opposite edges of the square, we can say that the edge length must be no longer than the distance between the two opposite points. (Say the two opposite points are distance d apart, and consider that they must lie on two different parallel lines that are distance p apart. The closest that two points from two different parallel lines that are a distance p apart could be is p, so if d is smaller than p then you can't draw two parallel lines containing those points.) Also, the edge length must be at least the distance between those opposite points divided by the square root of 2. (Think of a square where the two opposite points are very near opposite vertices of the square, if the square were any smaller then it would not be able to touch both points.) For a set of four points, there are two pairs of opposite points and we can call the distances between the pairs d1 and d2, placing limits on the square's edge length e such that d1/sqrt(2) < e <= d1 and d2/sqrt(2) < e <= d2. If you define d1 as the shorter of the two diagonals, this means that d2/sqrt(2) < e <= d1, so d1 had better be greater than d2/sqrt(2).In summary: points must be convex and not concave, and the pairs of opposite points must have distances between them such that the longer distance is no greater than sqrt(2) times the shorter. Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted May 3, 2014 Author Report Share Posted May 3, 2014 Yes, clearly not every set of four points (no three of which are collinear) lie on a square. Let's limit the puzzle to a set of points similar to the ones in the OP. Using standard constructions (compass, ruler) can you draw a square that has these four points (one each) on its sides? Quote Link to comment Share on other sites More sharing options...
0 plasmid Posted May 3, 2014 Report Share Posted May 3, 2014 Yes, I believe you can.Consider the points (-1,0), (1,0), and any third point C that lies on the unit circle with an angle t from the x-axis going counterclockwise. The distance along the x-axis from (-1,0) to C is 1+cos(t) The distance along the x-axis from (1,0) to C is 1-cos(t) The distance along the y-axis from either (-1,0) or (1,0) to C is sin(t) The length of the line from (-1,0) to C is sqrt[(distance along x-axis)2 + (distance along y-axis)2] = sqrt[(1+cos(t))2 + (sin(t))2] = sqrt[1 + 2cos(t) + cos2(t) + sin2(t)] = sqrt[2 + 2cos(t)] The length of the line from (1,0) to C is sqrt[(1-cos(t))2 + (sin(t))2] = sqrt[1 - 2cos(t) + cos2(t) + sin2(t)] = sqrt[2 - 2cos(t)] The square root of [(length from (-1,0) to C) squared + (length from (1,0) to C) squared] = sqrt[(2 + 2cos(t)) + (2 - 2cos(t)] = sqrt[4] = 2 which is equal to the distance between (-1,0) and (1,0) This proves that the angle from (-1,0) to C to (1,0) forms a right angle if C is on the unit circle, and is a useful fact to deduce the answer. Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted May 4, 2014 Author Report Share Posted May 4, 2014 By modifying the OP slightly I believe the four points may be completely arbitrary: Take any four points A, B, C, D in the plane, no three of which are collinear. They describe a unique quadrilateral, if we take the points as being its vertices. But they also describe a square, if we require only that the points lie on its sides or their extensions. The OP did not state, but it implied, that A B C D are taken in sequence. That is, the sides assiciated with A and C are opposite sides, (and so also with B and D) for the construction to be unique. That is, any four points, no three collinear, define two unique pairs of parallel lines, one point on each line, that intersect to form a unique square. Find a compass - ruler construction for those four lines. Quote Link to comment Share on other sites More sharing options...
0 plasmid Posted May 4, 2014 Report Share Posted May 4, 2014 There's one more exception of points that can't be squared, proof of which would require giving my answer to the problem, so here goes. Consider two diagonally opposite points, A and C, and draw a circle with those points at opposite ends of its diameter. One of my earlier posts pointed out that A and C must lie on opposite (parallel) lines of the square, so the solution must have two parallel lines of the square going through A and C. Consider the following figure - if those two parallel lines are not at 90 degrees to line AC, then they will intersect the circle at two points, once at point A or C and once at some other points which I'll call X and Y. Angle A-Y-C is a right angle, which I've proven in a previous post, meaning that line AY is the length of the edges of the square. In the next figure, the red circle is essentially the same as the black circle in the figure above, except that it's been moved to the right. Specifically, the red circle is drawn such that its perimeter includes the intersection of lines AC and BD, and its center is on the (infinite) line AC. Based on the previous paragraph, if you were to draw a line from the intersection of AC BD to another point on the red circle, then its length would be the edge length of the square if you were to draw lines of the square at that angle. If you do the same thing with points B and D, that will produce the blue circle. Now what we need to find are two lines, one going from the AC BD intersection to the red circle and one going from the AC BD intersection to the blue circle, which are perpendicular and equal in length so they would produce a square. In order to pull that off with a compass and straight edge, you can rotate the blue circle 90 degrees around the AC BD intersection, which creates the orange circle in the figure. Then the red circle and orange circle will intersect at one point* labeled E, and the line from the AC BD intersection to point E will be the length of the square's edges and will be parallel to the square's lines going through points B and D. It would then be straightforward to use that line to construct the square. I did not walk through how to actually do all that with a compass and straight edge, but it can all be done. *Footnote: This is the exception I alluded to before the spoiler. If the red circle and the orange circle have no intersection (aside from the AC BD intersection itself), then there will be no solution. That will be the case if and only if AC and BD are perpendicular and of different lengths. Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted May 19, 2014 Author Report Share Posted May 19, 2014 Two perpendicular line segments of equal length can have their respective endpoints lie on the four sides of a square, or their extensions. Use two nonadjacent points, of the four given, to define one of the line segments. drop a perpendicular of equal length from a third point and take it from there. Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted June 6, 2014 Author Report Share Posted June 6, 2014 (edited) Starting with Draw AC, and find BD' as shown, where BD' = AC. D and D' are on one side of the square, (or its extension.) Draw that line and drop perpendiculars to it through A and C. Finally, draw a perpendicular to both of them through B. The result is the red square. Plasmid, does this cover the problem cases you noted? Edited June 9, 2014 by bonanova Added the final figure to the solution Quote Link to comment Share on other sites More sharing options...
0 plasmid Posted June 7, 2014 Report Share Posted June 7, 2014 If lines AC and BD are perpendicular and of different length, then line D-D' will not have two different perpendiculars with one going through A and one going through C... both perpendiculars will emanate from the same point. So in the end it wouldn't really describe a square so much as a point whose "edges" if extended would touch each of the four points.But I do think your solution is far more Aha-y. 1 Quote Link to comment Share on other sites More sharing options...
0 bonanova Posted June 7, 2014 Author Report Share Posted June 7, 2014 Good point. (no pun intended.) That would be a degenerate case - a square with side zero. And it wouldn't help to draw BD first. You'd get AC' collinear with C, with the same result. Quote Link to comment Share on other sites More sharing options...
Question
bonanova
Take any four points A, B, C, D in the plane, no three of which are collinear.
They describe a unique quadrilateral, if we take the points as being its vertices.
But they also describe a square, if we require only that the points lie on its sides.
Using a compass and straightedge, construct a square such that the four points lie, one each, on its sides
Edit: or the extensions of its sides.
. . . . . . . . . . . . . . .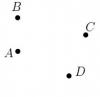
Hint: The points are not special.
Draw four similar points and do the construction on a sheet of paper if that helps.
The answer would then be to describe the process.
Edited by bonanovaRelax the constraints
Link to comment
Share on other sites
11 answers to this question
Recommended Posts
Join the conversation
You can post now and register later. If you have an account, sign in now to post with your account.